Valore assoluto
Definizione
Il valore assoluto è una funzione reale di variabile reale,
[math]|cdot|: mathbb{R} o mathbb{R}[/math]
, che associa alla variabile [math]x[/math]
il numero stesso se [math]x[/math]
è non negativa, [math]-x[/math]
se invece [math]x[/math]
è negativa. Il valore assoluto di [math]x[/math]
si indica con [math]|x|[/math]
, e risulta
[math]|x| = \begin{cases} x & quad \text{se } x ge 0 \\ -x & quad \text{se } x < 0 \ \end{cases}[/math]
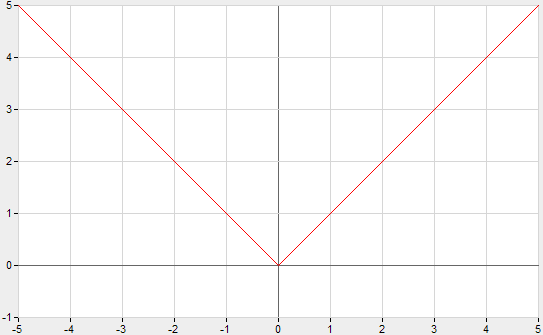
Di seguito viene riportato il grafico della funzione valore assoluto.
Proprietà del valore assoluto
Il valore assoluto è una funzione definita positiva, in quanto gode delle due seguenti proprietà
[math]|x| ge 0 quad forall x in mathbb{R}[/math]
[math]|x| = 0 iff x = 0[/math]
Il valore assoluto è anche una funzione positivamente omogenea, infatti
[math]|x cdot y| = |x| cdot |y| quad forall x, y in mathbb{R}[/math]
[math]|frac{x}{y}| = frac{|x|}{|y|} quad forall x in mathbb{R}, quad forall y in mathbb{R} setmi
us {0}[/math]
us {0}[/math]
Vale anche la disuguaglianza traingolare, ovvero
[math]|x + y| le |x| + |y| quad forall x, y in mathbb{R}[/math]
Grazie a queste tre condizioni si può affermare che il valore assoluto è una norma. Conseguenza diretta della disuguaglianza triangolare è la seguente
[math]||x| - |y|| le |x - y| quad forall x, y in mathbb{R}[/math]
Inoltre, per ogni
[math]n in mathbb{N}[/math]
pari, risulta
[math]
oot{n}{x^n} = |x| quad forall x in mathbb{R}[/math]
oot{n}{x^n} = |x| quad forall x in mathbb{R}[/math]
Le seguenti proprietà, utili per la risoluzione di equazioni e disequazioni con valori assoluti, sono conseguenza diretta della definizione
[math]|x| = |c| implies x = \pm c[/math]
[math]|x| = c implies x = \pm c[/math]
[math]|x| le c implies {(
exists x in mathbb\begin{cases} & quad \text{se } c < 0 \\ x = 0 & quad \text{se } c = 0 \\ -c le x le c & quad \text{se } c > 0 \ \end{cases}[/math]
exists x in mathbb\begin{cases} & quad \text{se } c < 0 \\ x = 0 & quad \text{se } c = 0 \\ -c le x le c & quad \text{se } c > 0 \ \end{cases}[/math]
[math]|x| < c implies {(
exists x in mathbb\begin{cases} & quad \text{se } c le 0 \\ -c < x < c & quad \text{se } c > 0 \ \end{cases}[/math]
exists x in mathbb\begin{cases} & quad \text{se } c le 0 \\ -c < x < c & quad \text{se } c > 0 \ \end{cases}[/math]
[math]|x| ge c implies \begin{cases} x in mathbb{R} & quad \text{se } c le 0 \\ x le -c vee x ge c & quad \text{se } c > 0 \ \end{cases}[/math]
[math]|x| > c implies {(x in mathbb{R}, quad \text{se } c < 0),(x
e 0, quad \text\egin{cases} & se } c = 0 \\ x < -c vee x > c & quad \text{se } c > 0 \ \end{cases}[/math]
e 0, quad \text\egin{cases} & se } c = 0 \\ x < -c vee x > c & quad \text{se } c > 0 \ \end{cases}[/math]
Infine il valore assoluto di un numero può anche essere espresso per mezzo del massimo fra
[math]x[/math]
e [math]-x[/math]
[math]|x| = max {x, -x} quad forall x in mathbb{R}[/math]
Funzione segno
Definizione
La funzione segno è una funzione reale di variabile reale, [math]\text{sgn}: mathbb{R} o mathbb{R}[/math], che vale [math]1[/math] quando il suo argomento è positivo, [math]-1[/math] quando il suo argomento è negativo, [math]0[/math] atrimenti. In formule
[math]\text\egin{cases} & quad \text{se } x > 0 \\ 0 & quad \text{se } x = 0 \\ -1 & quad \text{se } x < 0 \ \end{cases}[/math]
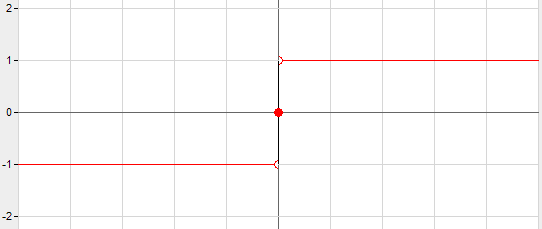
Di seguito viene riportato il grafico della funzione segno.
Proprietà della funzione segno
Proprietà della funzione segno
[math]|x| = x cdot \text{sgn}(x) quad forall x in mathbb{R}[/math]
[math]\text{sgn}(x) = frac{|x|}{x} = frac{x}{|x|} quad forall x in mathbb{R} setmi
us {0}[/math]
us {0}[/math]
Parte intera
Parte intera
Definizione
Dato un numero reale
[math]x[/math]
, si definisce parte intera superiore di [math]x[/math]
, e si indica con [math]lceil x
ceil[/math]
, il più piccolo intero non minore di ceil[/math]
[math]x[/math]
. Analogamente si indica la parte intera inferiore di [math]x[/math]
come il più grande intero minore o uguale di [math]x[/math]
, e si indica con [math]lfloor x
floor[/math]
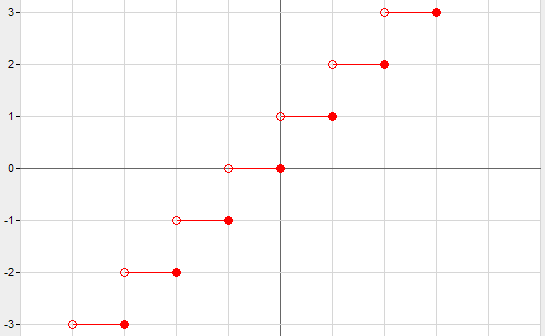
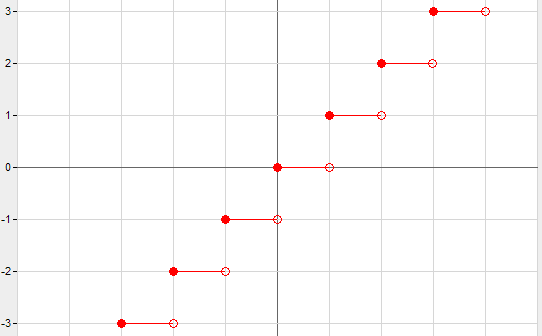
. Sono riportati di seguito i grafici delle funzioni parte intera superiore e inferiore, rispettivamente.floor[/math]
Proprietà della parte intera
Proprietà
[math]lfloor x
floor = x = lceil x
ceil iff x in mathbb{Z}[/math]
floor = x = lceil x
ceil iff x in mathbb{Z}[/math]
[math]lfloor lfloor x
floor
floor = lfloor x
floor quad forall x in mathbb{R}[/math]
floor
floor = lfloor x
floor quad forall x in mathbb{R}[/math]
[math]lceil lceil x
ceil
ceil = lceil x
ceil quad forall x in mathbb{R}[/math]
ceil
ceil = lceil x
ceil quad forall x in mathbb{R}[/math]
[math]lfloor x + y
floor = x + lfloor y
floor quad forall (x,y) in mathbb{Z} imes mathbb{R}[/math]
floor = x + lfloor y
floor quad forall (x,y) in mathbb{Z} imes mathbb{R}[/math]
[math]lceil x + y
ceil = x + lceil y
ceil quad forall (x,y) in mathbb{Z} imes mathbb{R}[/math]
ceil = x + lceil y
ceil quad forall (x,y) in mathbb{Z} imes mathbb{R}[/math]
[math]lfloor x
floor le x < lfloor x
floor + 1 quad forall x in mathbb{R}[/math]
floor le x < lfloor x
floor + 1 quad forall x in mathbb{R}[/math]
[math]x le lceil x
ceil < x + 1 quad forall x in mathbb{R}[/math]
ceil < x + 1 quad forall x in mathbb{R}[/math]
[math]lceil x
ceil = - lfloor -x
floor quad forall x in mathbb{R}[/math]
ceil = - lfloor -x
floor quad forall x in mathbb{R}[/math]
[math]x = lfloor frac{x}{2}
floor + lceil frac{x}{2}
ceil quad forall x in mathbb{Z}[/math]
floor + lceil frac{x}{2}
ceil quad forall x in mathbb{Z}[/math]
Infine, se
[math]m[/math]
e [math]n[/math]
sono due interi primi fra di loro, risulta
[math]\sum_{i=1}^{n-1} lfloor i frac{m}{n}
floor = frac{(m-1)(n-1)}{2}[/math]
floor = frac{(m-1)(n-1)}{2}[/math]

